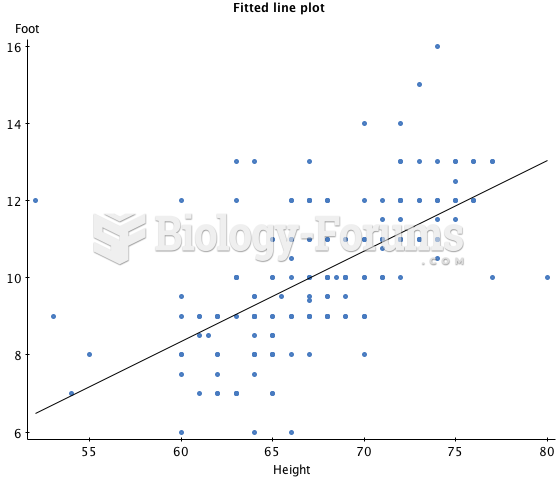
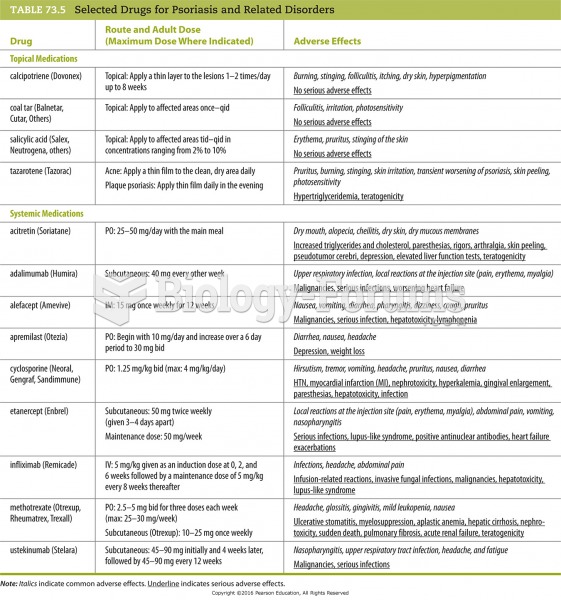
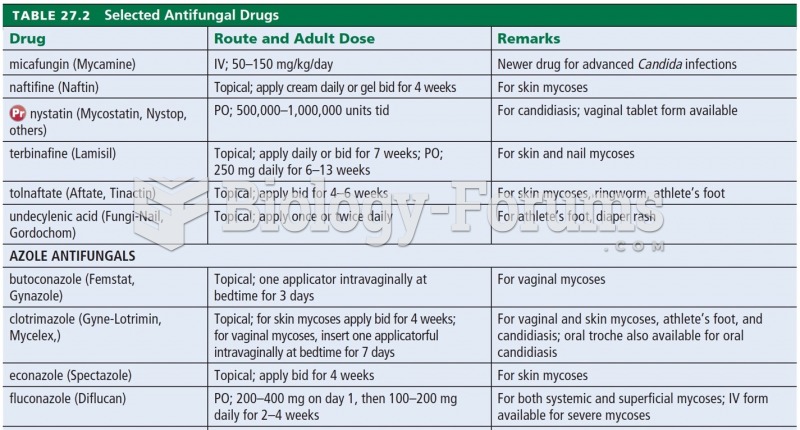
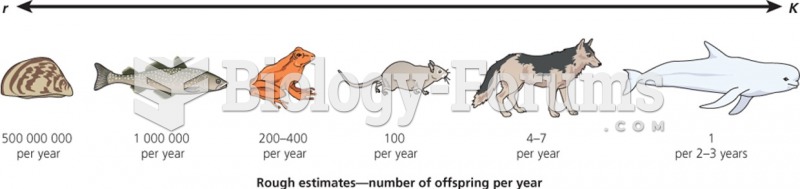
|
|
|
The U.S. Preventive Services Task Force recommends that all women age 65 years of age or older should be screened with bone densitometry.
ACTH levels are normally highest in the early morning (between 6 and 8 A.M.) and lowest in the evening (between 6 and 11 P.M.). Therefore, a doctor who suspects abnormal levels looks for low ACTH in the morning and high ACTH in the evening.
Everyone has one nostril that is larger than the other.
As of mid-2016, 18.2 million people were receiving advanced retroviral therapy (ART) worldwide. This represents between 43–50% of the 34–39.8 million people living with HIV.
Immunoglobulin injections may give short-term protection against, or reduce severity of certain diseases. They help people who have an inherited problem making their own antibodies, or those who are having certain types of cancer treatments.