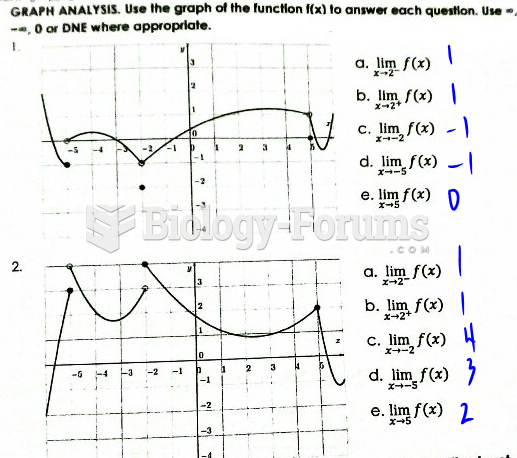
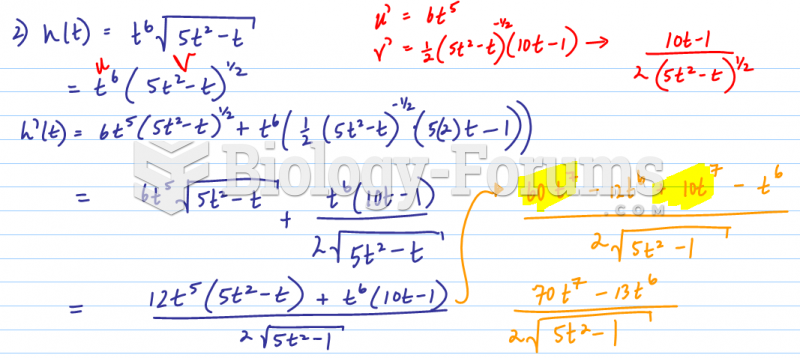
|
|
|
Over time, chronic hepatitis B virus and hepatitis C virus infections can progress to advanced liver disease, liver failure, and hepatocellular carcinoma. Unlike other forms, more than 80% of hepatitis C infections become chronic and lead to liver disease. When combined with hepatitis B, hepatitis C now accounts for 75% percent of all cases of liver disease around the world. Liver failure caused by hepatitis C is now leading cause of liver transplants in the United States.
The Food and Drug Administration has approved Risperdal, an adult antipsychotic drug, for the symptomatic treatment of irritability in children and adolescents with autism. The approval is the first for the use of a drug to treat behaviors associated with autism in children. These behaviors are included under the general heading of irritability and include aggression, deliberate self-injury, and temper tantrums.
The first successful kidney transplant was performed in 1954 and occurred in Boston. A kidney from an identical twin was transplanted into his dying brother's body and was not rejected because it did not appear foreign to his body.
Women are two-thirds more likely than men to develop irritable bowel syndrome. This may be attributable to hormonal changes related to their menstrual cycles.
The most common childhood diseases include croup, chickenpox, ear infections, flu, pneumonia, ringworm, respiratory syncytial virus, scabies, head lice, and asthma.