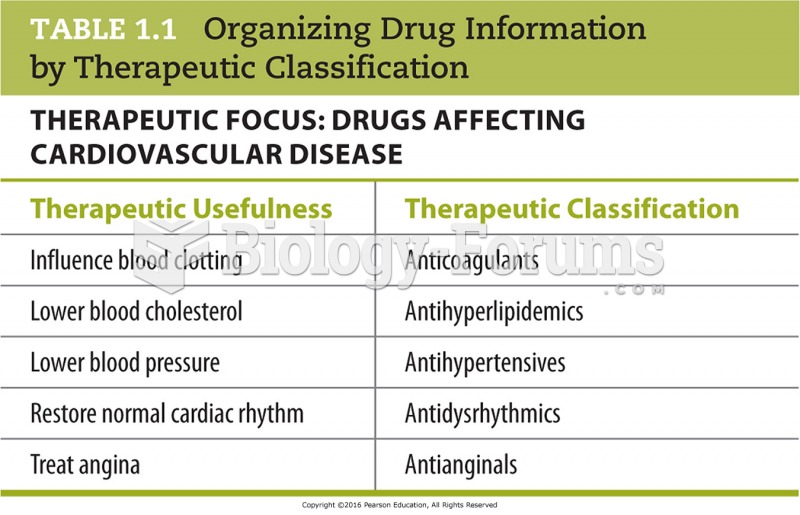
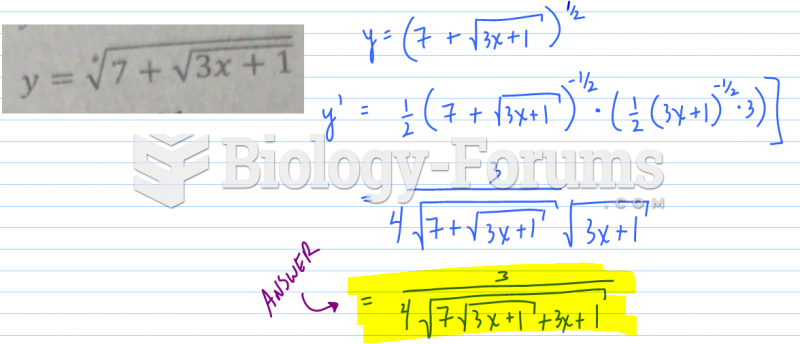
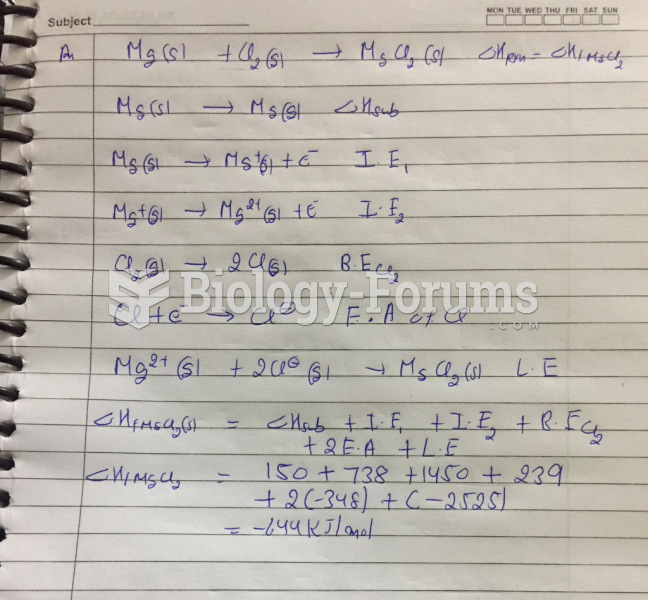
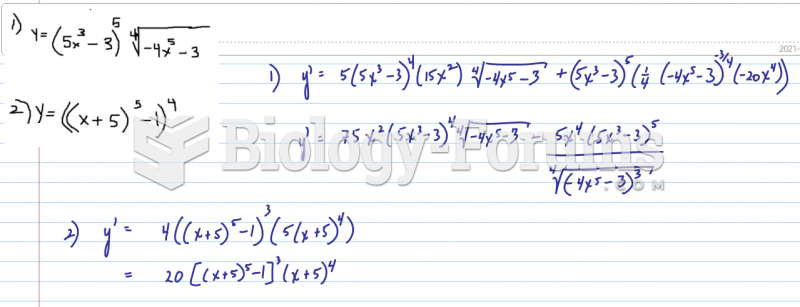|
|
|
More than 20 million Americans cite use of marijuana within the past 30 days, according to the National Survey on Drug Use and Health (NSDUH). More than 8 million admit to using it almost every day.
Your skin wrinkles if you stay in the bathtub a long time because the outermost layer of skin (which consists of dead keratin) swells when it absorbs water. It is tightly attached to the skin below it, so it compensates for the increased area by wrinkling. This happens to the hands and feet because they have the thickest layer of dead keratin cells.
The largest baby ever born weighed more than 23 pounds but died just 11 hours after his birth in 1879. The largest surviving baby was born in October 2009 in Sumatra, Indonesia, and weighed an astounding 19.2 pounds at birth.
A strange skin disease referred to as Morgellons has occurred in the southern United States and in California. Symptoms include slowly healing sores, joint pain, persistent fatigue, and a sensation of things crawling through the skin. Another symptom is strange-looking, threadlike extrusions coming out of the skin.
The first oncogene was discovered in 1970 and was termed SRC (pronounced "SARK").