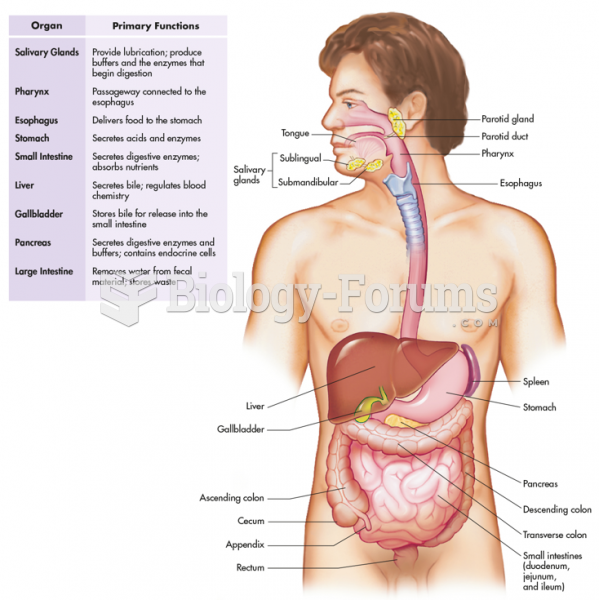
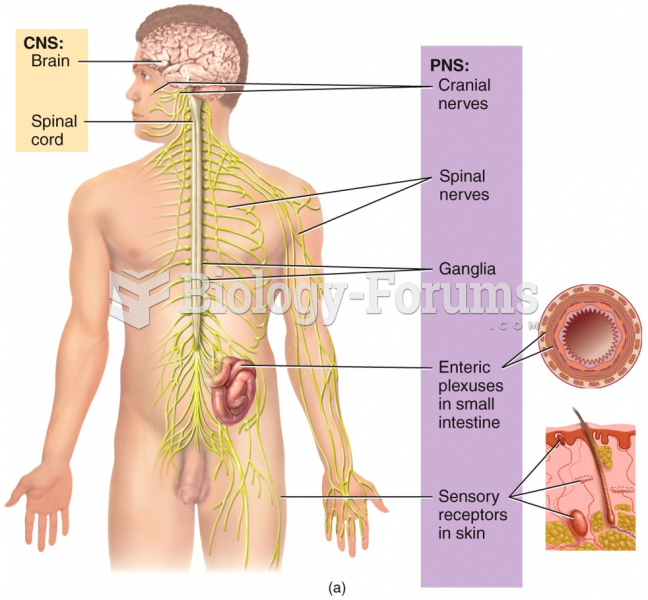
|
|
|
It is widely believed that giving a daily oral dose of aspirin to heart attack patients improves their chances of survival because the aspirin blocks the formation of new blood clots.
Certain rare plants containing cyanide include apricot pits and a type of potato called cassava. Fortunately, only chronic or massive ingestion of any of these plants can lead to serious poisoning.
The average older adult in the United States takes five prescription drugs per day. Half of these drugs contain a sedative. Alcohol should therefore be avoided by most senior citizens because of the dangerous interactions between alcohol and sedatives.
Addicts to opiates often avoid treatment because they are afraid of withdrawal. Though unpleasant, with proper management, withdrawal is rarely fatal and passes relatively quickly.
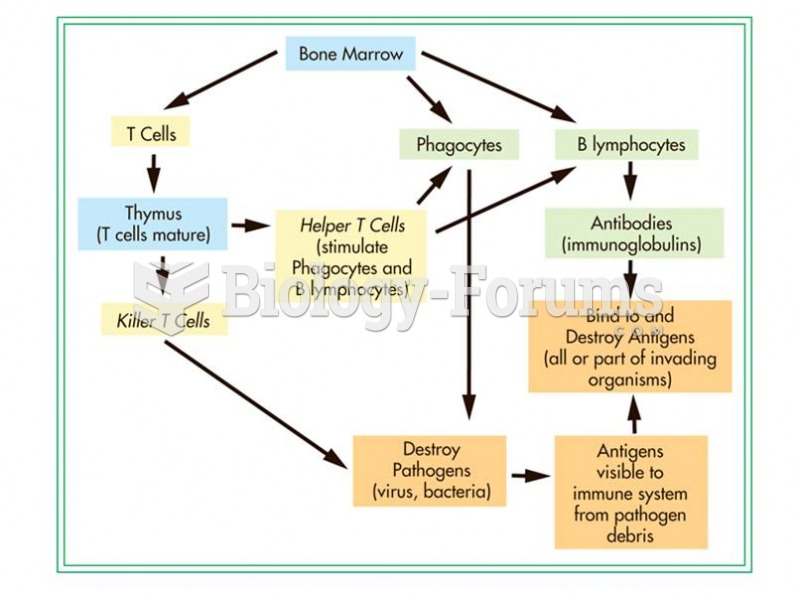
Autoimmune diseases occur when the immune system destroys its own healthy tissues. When this occurs, white blood cells cannot distinguish between pathogens and normal cells.