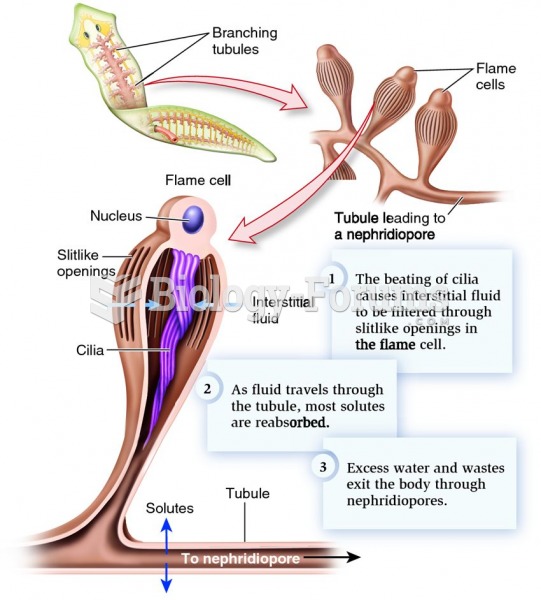
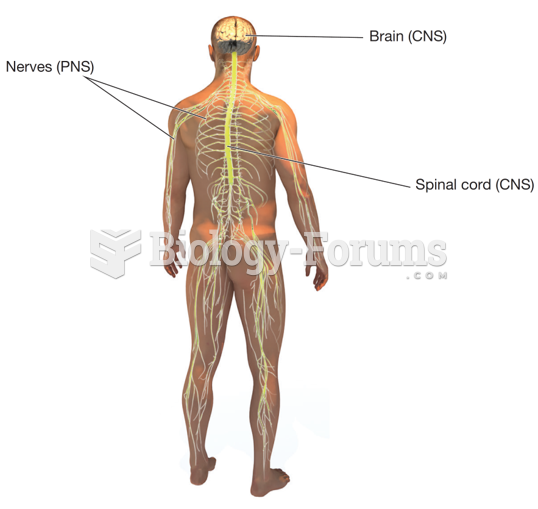
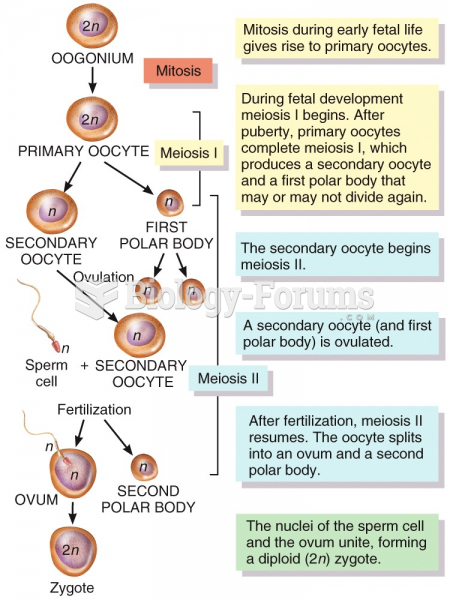
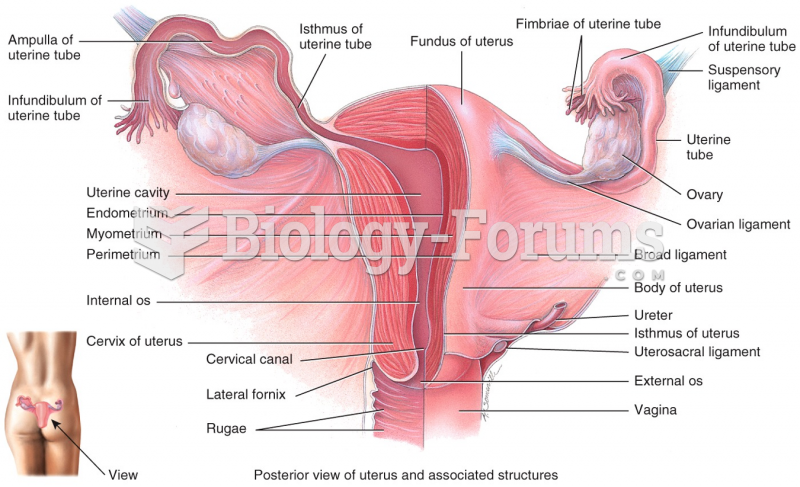
|
|
|
Bacteria have been found alive in a lake buried one half mile under ice in Antarctica.
Adolescents often feel clumsy during puberty because during this time of development, their hands and feet grow faster than their arms and legs do. The body is therefore out of proportion. One out of five adolescents actually experiences growing pains during this period.
Hypertension is a silent killer because it is deadly and has no significant early symptoms. The danger from hypertension is the extra load on the heart, which can lead to hypertensive heart disease and kidney damage. This occurs without any major symptoms until the high blood pressure becomes extreme. Regular blood pressure checks are an important method of catching hypertension before it can kill you.
The U.S. Pharmacopeia Medication Errors Reporting Program states that approximately 50% of all medication errors involve insulin.
Each year in the United States, there are approximately six million pregnancies. This means that at any one time, about 4% of women in the United States are pregnant.