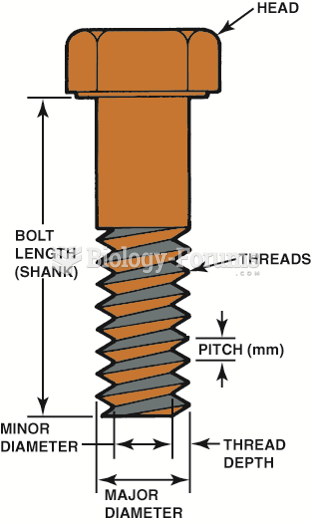
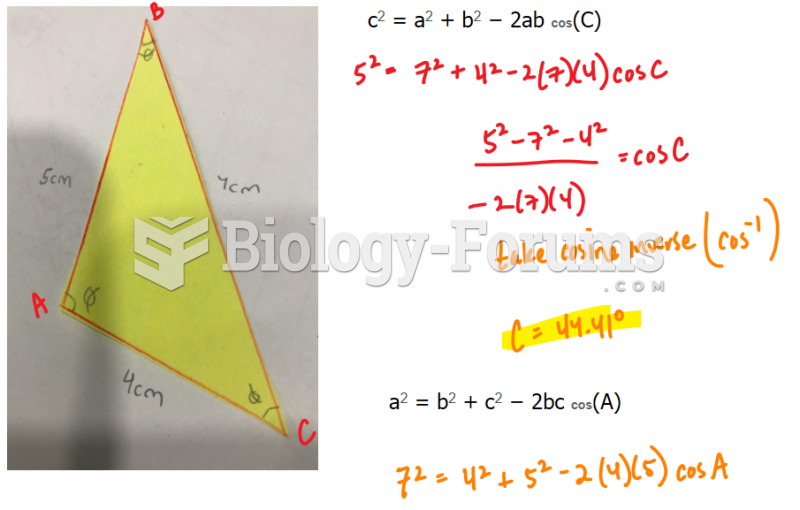
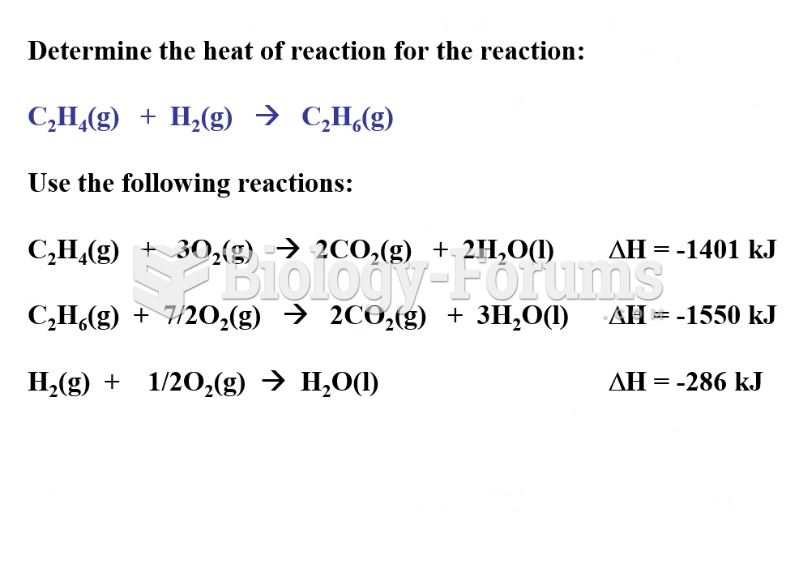
|
|
|
Stevens-Johnson syndrome and Toxic Epidermal Necrolysis syndrome are life-threatening reactions that can result in death. Complications include permanent blindness, dry-eye syndrome, lung damage, photophobia, asthma, chronic obstructive pulmonary disease, permanent loss of nail beds, scarring of mucous membranes, arthritis, and chronic fatigue syndrome. Many patients' pores scar shut, causing them to retain heat.
Opium has influenced much of the world's most popular literature. The following authors were all opium users, of varying degrees: Lewis Carroll, Charles, Dickens, Arthur Conan Doyle, and Oscar Wilde.
IgA antibodies protect body surfaces exposed to outside foreign substances. IgG antibodies are found in all body fluids. IgM antibodies are the first type of antibody made in response to an infection. IgE antibody levels are often high in people with allergies. IgD antibodies are found in tissues lining the abdomen and chest.
The familiar sounds of your heart are made by the heart's valves as they open and close.
The average adult has about 21 square feet of skin.