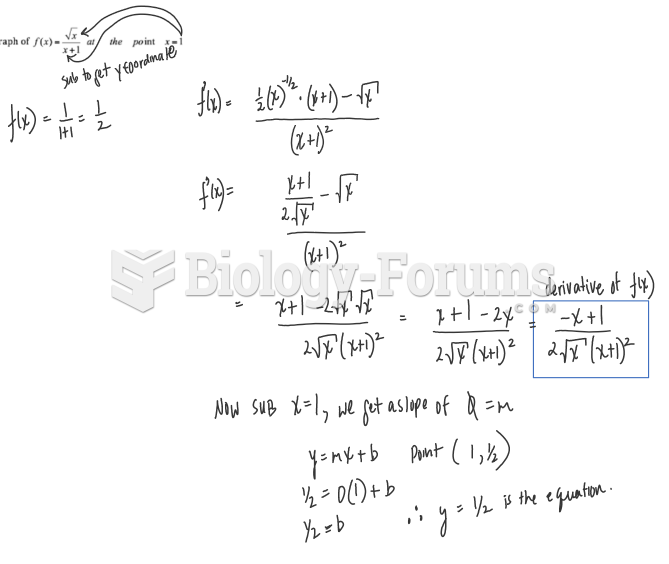
|
|
|
The Babylonians wrote numbers in a system that used 60 as the base value rather than the number 10. They did not have a symbol for "zero."
More than one-third of adult Americans are obese. Diseases that kill the largest number of people annually, such as heart disease, cancer, diabetes, stroke, and hypertension, can be attributed to diet.
According to the American College of Allergy, Asthma & Immunology, more than 50 million Americans have some kind of food allergy. Food allergies affect between 4 and 6% of children, and 4% of adults, according to the CDC. The most common food allergies include shellfish, peanuts, walnuts, fish, eggs, milk, and soy.
Acute bronchitis is an inflammation of the breathing tubes (bronchi), which causes increased mucus production and other changes. It is usually caused by bacteria or viruses, can be serious in people who have pulmonary or cardiac diseases, and can lead to pneumonia.
Approximately one in four people diagnosed with diabetes will develop foot problems. Of these, about one-third will require lower extremity amputation.