|
|
|
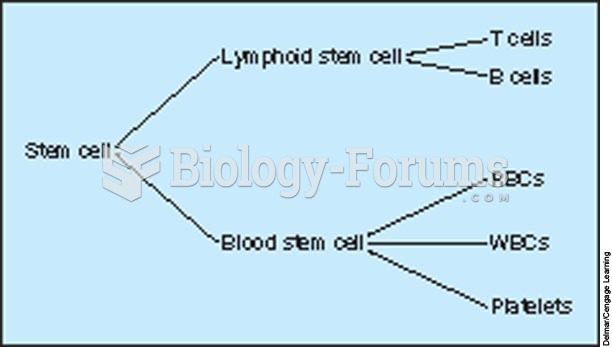
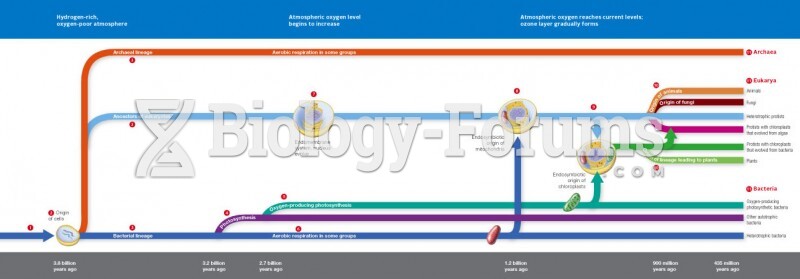
The term bacteria was devised in the 19th century by German biologist Ferdinand Cohn. He based it on the Greek word "bakterion" meaning a small rod or staff. Cohn is considered to be the father of modern bacteriology.
Carbamazepine can interfere with the results of home pregnancy tests. If you are taking carbamazepine, do not try to test for pregnancy at home.
The use of salicylates dates back 2,500 years to Hippocrates's recommendation of willow bark (from which a salicylate is derived) as an aid to the pains of childbirth. However, overdosage of salicylates can harm body fluids, electrolytes, the CNS, the GI tract, the ears, the lungs, the blood, the liver, and the kidneys and cause coma or death.
In inpatient settings, adverse drug events account for an estimated one in three of all hospital adverse events. They affect approximately 2 million hospital stays every year, and prolong hospital stays by between one and five days.
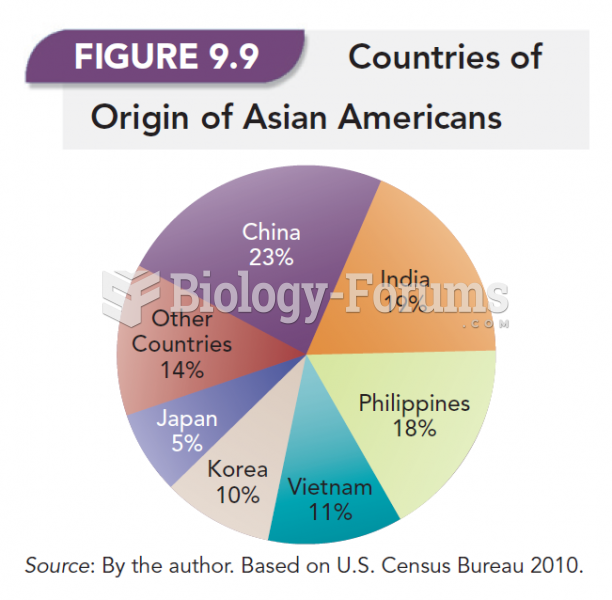
The senior population grows every year. Seniors older than 65 years of age now comprise more than 13% of the total population. However, women outlive men. In the 85-and-over age group, there are only 45 men to every 100 women.