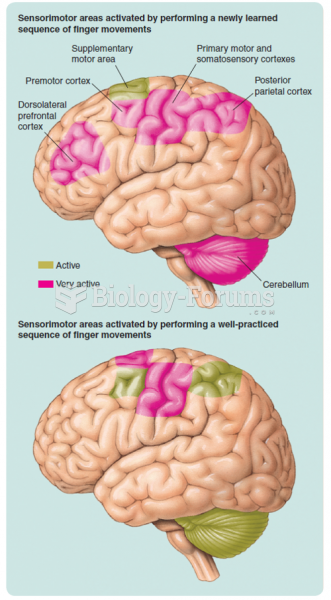
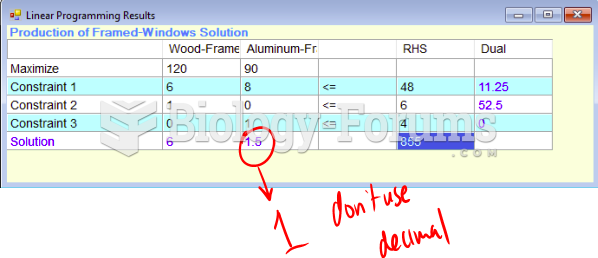
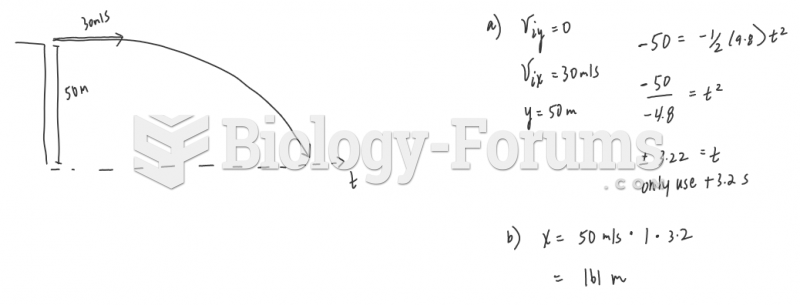|
|
|
In the United States, there is a birth every 8 seconds, according to the U.S. Census Bureau's Population Clock.
In 1844, Charles Goodyear obtained the first patent for a rubber condom.
About 80% of major fungal systemic infections are due to Candida albicans. Another form, Candida peritonitis, occurs most often in postoperative patients. A rare disease, Candida meningitis, may follow leukemia, kidney transplant, other immunosuppressed factors, or when suffering from Candida septicemia.
Signs of depression include feeling sad most of the time for 2 weeks or longer; loss of interest in things normally enjoyed; lack of energy; sleep and appetite disturbances; weight changes; feelings of hopelessness, helplessness, or worthlessness; an inability to make decisions; and thoughts of death and suicide.
A cataract is a clouding of the eyes' natural lens. As we age, some clouding of the lens may occur. The first sign of a cataract is usually blurry vision. Although glasses and other visual aids may at first help a person with cataracts, surgery may become inevitable. Cataract surgery is very successful in restoring vision, and it is the most frequently performed surgery in the United States.