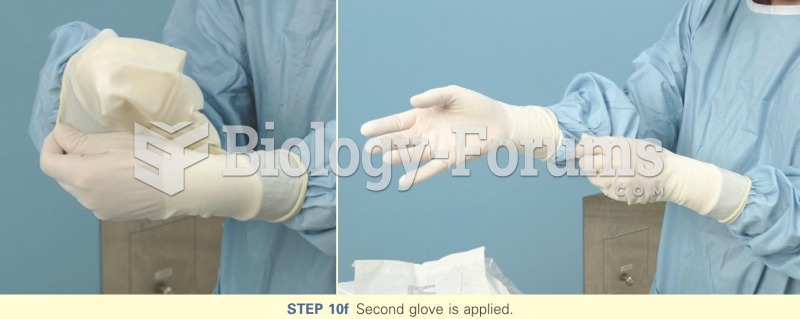
This topic contains a solution. Click here to go to the answer
|
|
|
Did you know?
Malaria was not eliminated in the United States until 1951. The term eliminated means that no new cases arise in a country for 3 years.
Did you know?
According to the FDA, adverse drug events harmed or killed approximately 1,200,000 people in the United States in the year 2015.
Did you know?
When blood is exposed to air, it clots. Heparin allows the blood to come in direct contact with air without clotting.
Did you know?
In the United States, there is a birth every 8 seconds, according to the U.S. Census Bureau's Population Clock.
Did you know?
There are more sensory neurons in the tongue than in any other part of the body.