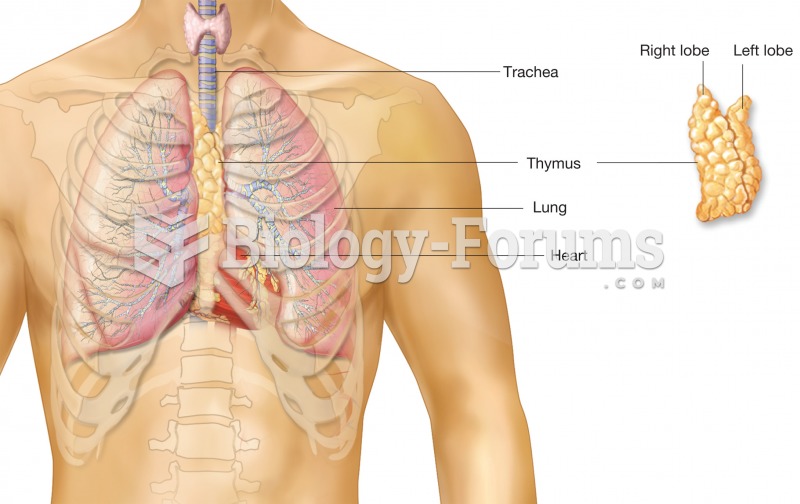
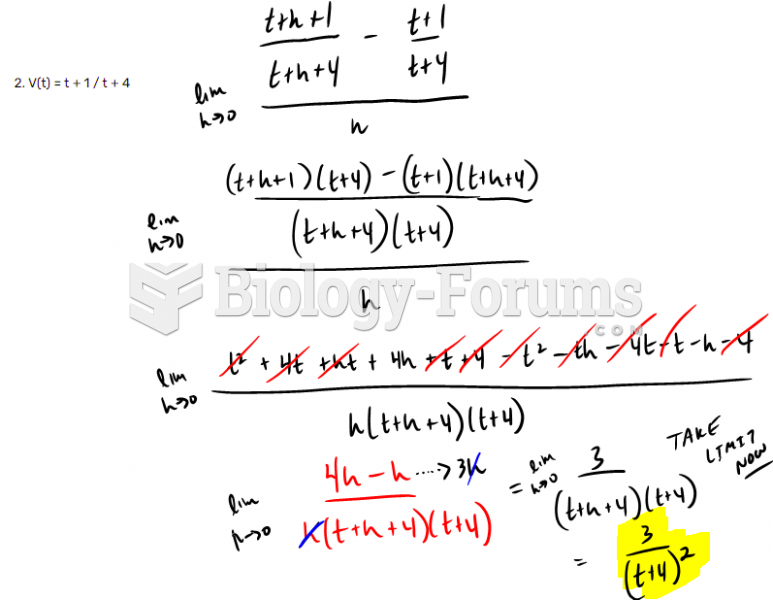
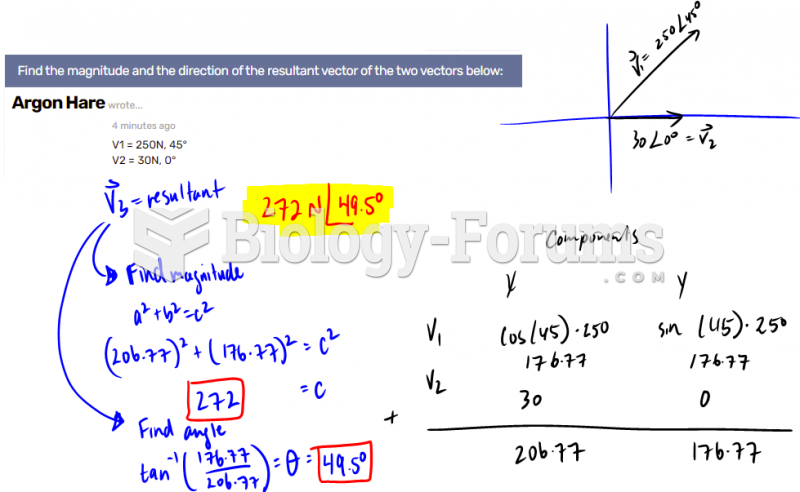
- Grade 11 and 12 Mathematics (Moderator: geoffrey)
|
|
|
- Grade 11 and 12 Mathematics (Moderator: geoffrey)
About 600,000 particles of skin are shed every hour by each human. If you live to age 70 years, you have shed 105 pounds of dead skin.
There are more sensory neurons in the tongue than in any other part of the body.
Inotropic therapy does not have a role in the treatment of most heart failure patients. These drugs can make patients feel and function better but usually do not lengthen the predicted length of their lives.
Hippocrates noted that blood separates into four differently colored liquids when removed from the body and examined: a pure red liquid mixed with white liquid material with a yellow-colored froth at the top and a black substance that settles underneath; he named these the four humors (for blood, phlegm, yellow bile, and black bile).
Dogs have been used in studies to detect various cancers in human subjects. They have been trained to sniff breath samples from humans that were collected by having them breathe into special tubes. These people included 55 lung cancer patients, 31 breast cancer patients, and 83 cancer-free patients. The dogs detected 54 of the 55 lung cancer patients as having cancer, detected 28 of the 31 breast cancer patients, and gave only three false-positive results (detecting cancer in people who didn't have it).