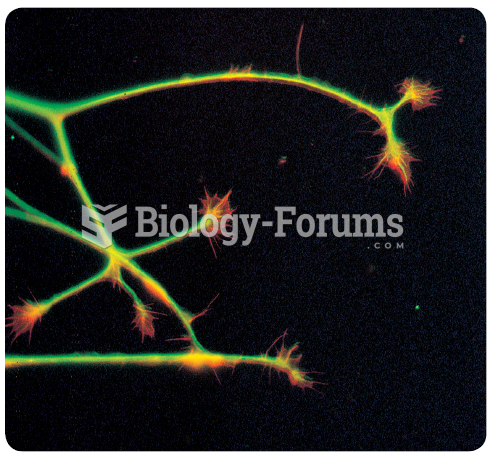
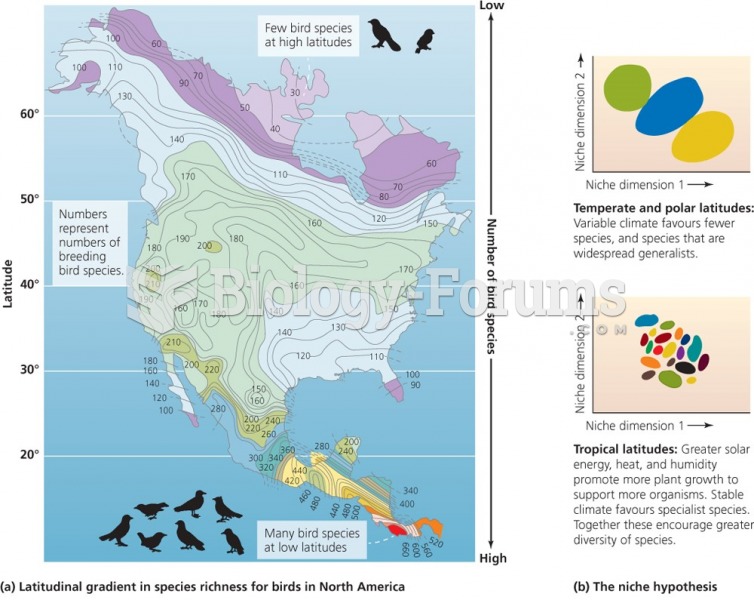
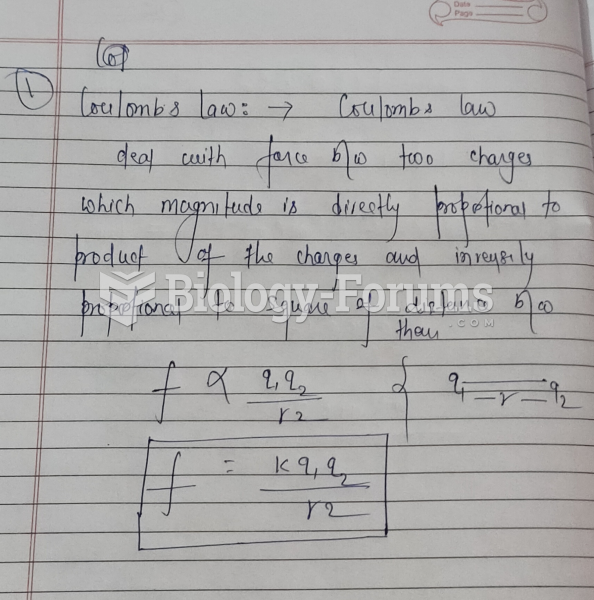
|
|
|
Asthma-like symptoms were first recorded about 3,500 years ago in Egypt. The first manuscript specifically written about asthma was in the year 1190, describing a condition characterized by sudden breathlessness. The treatments listed in this manuscript include chicken soup, herbs, and sexual abstinence.
Urine turns bright yellow if larger than normal amounts of certain substances are consumed; one of these substances is asparagus.
Persons who overdose with cardiac glycosides have a better chance of overall survival if they can survive the first 24 hours after the overdose.
All adverse reactions are commonly charted in red ink in the patient's record and usually are noted on the front of the chart. Failure to follow correct documentation procedures may result in malpractice lawsuits.
 If the valve clearance (lash) is not correct, loosen the retaining nut and turn the valve adjusting ...
If the valve clearance (lash) is not correct, loosen the retaining nut and turn the valve adjusting ...
 Each of the eight injectors shown are producing a correct spray pattern for the applications. While ...
Each of the eight injectors shown are producing a correct spray pattern for the applications. While ...