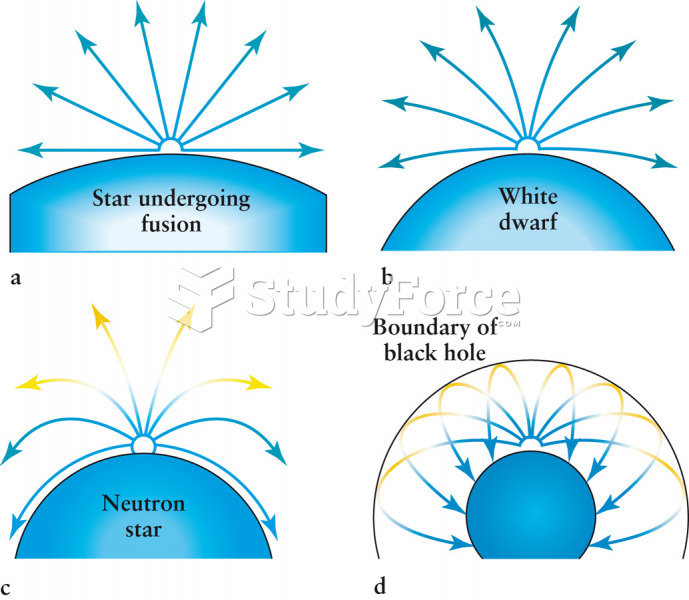
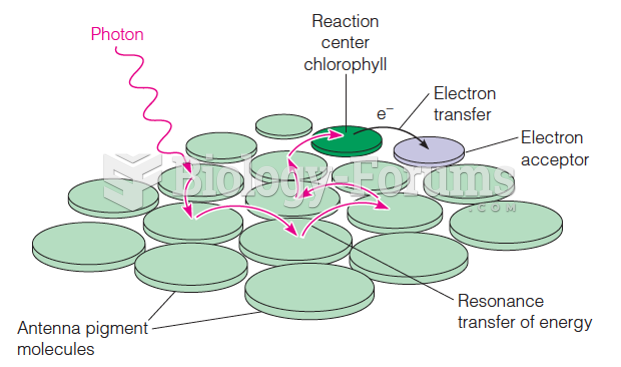
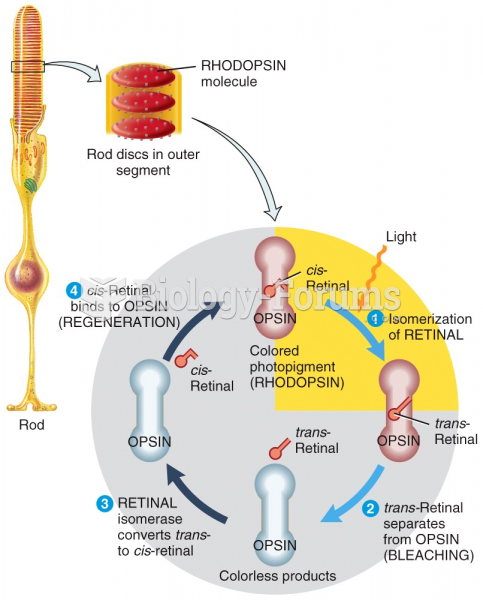
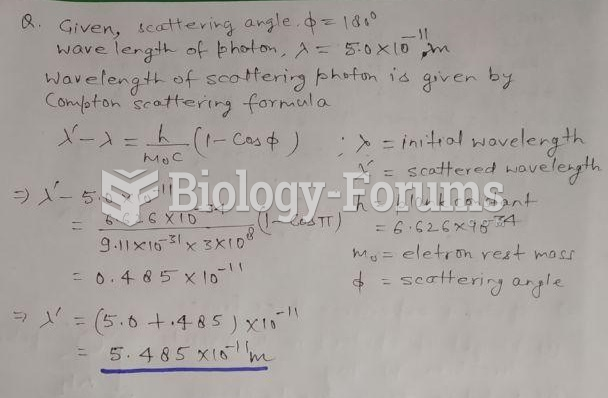
This topic contains a solution. Click here to go to the answer
|
|
|
Did you know?
It is difficult to obtain enough calcium without consuming milk or other dairy foods.
Did you know?
This year, an estimated 1.4 million Americans will have a new or recurrent heart attack.
Did you know?
Green tea is able to stop the scent of garlic or onion from causing bad breath.
Did you know?
ACTH levels are normally highest in the early morning (between 6 and 8 A.M.) and lowest in the evening (between 6 and 11 P.M.). Therefore, a doctor who suspects abnormal levels looks for low ACTH in the morning and high ACTH in the evening.
Did you know?
Approximately one in four people diagnosed with diabetes will develop foot problems. Of these, about one-third will require lower extremity amputation.