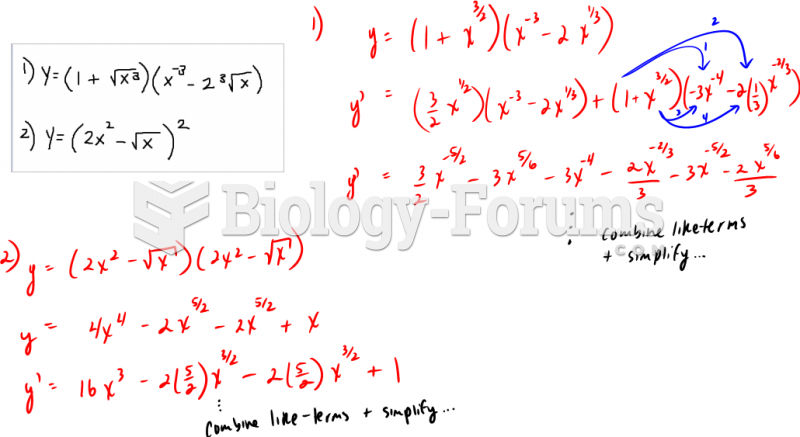
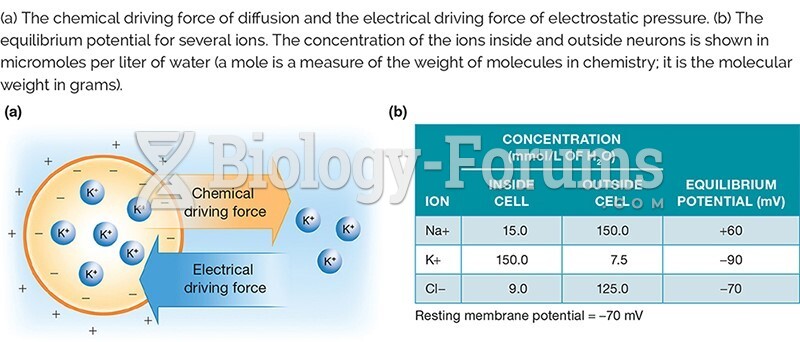
|
|
|
Adults are resistant to the bacterium that causes Botulism. These bacteria thrive in honey – therefore, honey should never be given to infants since their immune systems are not yet resistant.
The human body produces and destroys 15 million blood cells every second.
Colchicine is a highly poisonous alkaloid originally extracted from a type of saffron plant that is used mainly to treat gout.
Aspirin may benefit 11 different cancers, including those of the colon, pancreas, lungs, prostate, breasts, and leukemia.
As the western states of America were settled, pioneers often had to drink rancid water from ponds and other sources. This often resulted in chronic diarrhea, causing many cases of dehydration and death that could have been avoided if clean water had been available.