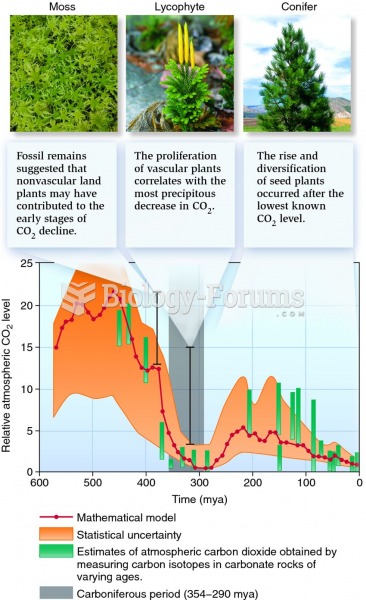
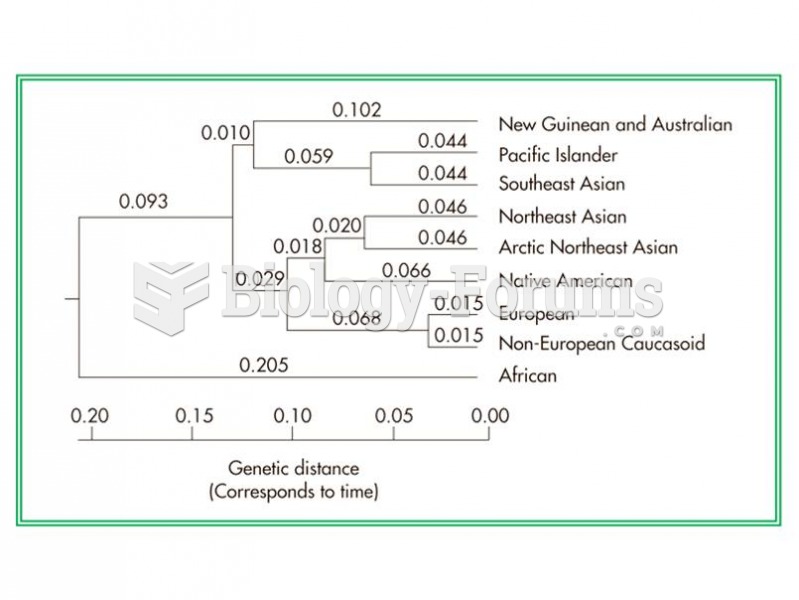
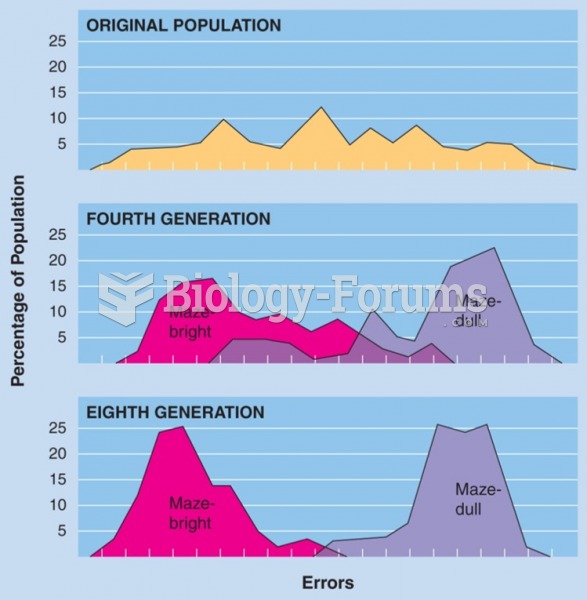
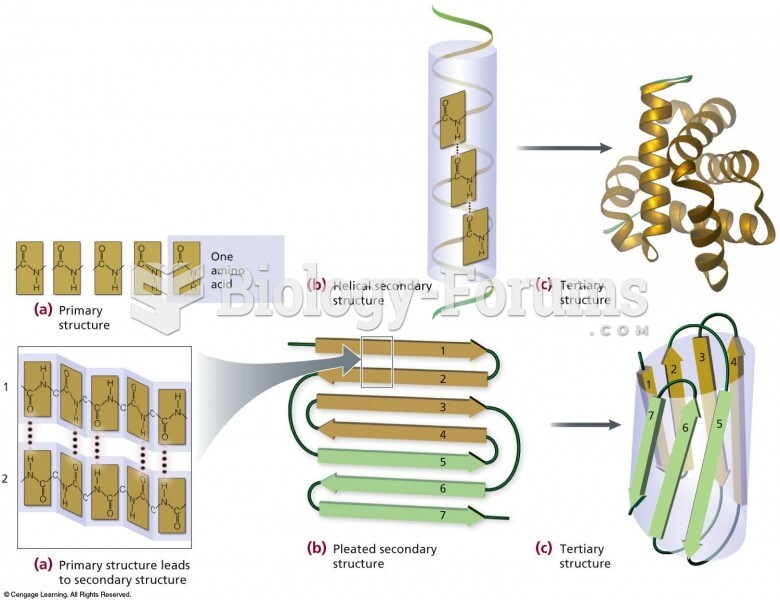
|
|
|
More than one-third of adult Americans are obese. Diseases that kill the largest number of people annually, such as heart disease, cancer, diabetes, stroke, and hypertension, can be attributed to diet.
Many of the drugs used by neuroscientists are derived from toxic plants and venomous animals (such as snakes, spiders, snails, and puffer fish).
According to the National Institute of Environmental Health Sciences, lung disease is the third leading killer in the United States, responsible for one in seven deaths. It is the leading cause of death among infants under the age of one year.
According to the CDC, approximately 31.7% of the U.S. population has high low-density lipoprotein (LDL) or "bad cholesterol" levels.
The modern decimal position system was the invention of the Hindus (around 800 AD), involving the placing of numerals to indicate their value (units, tens, hundreds, and so on).