This topic contains a solution. Click here to go to the answer
« previous next »

Author Question: Using the known geometric series representation = xn, valid for -1 < x < 1, find a power series ... (Read 97 times)

newbem

  • Hero Member
  • *****
  • Posts: 579
on: May 26, 2021
Using the known geometric series representation   = xn,  valid for  -1 < x < 1, find a power series representation for  f(x) = tan-1(2x)  in powers of  x. On what interval does the series converge to  f(x)?
◦ f(x) = ,  for  - < x ≤
◦ f(x) = ,  for  - ≤ x ≤
◦ f(x) = 2n + 1,  for  - 2 ≤ x ≤ 2
◦ f(x) = ,  for  - < x <
◦ f(x) = 2n + 1,  for  - 2 < x < 2
Calculus: A Complete Course ¦ Adams, Essex ¦ 8th Edition
Buy Test-Bank


Related Topics

Need homework help now?

Ask unlimited questions for free

Ask a Question
Marked as best answer by newbem on May 26, 2021

Smiles0805

  • Sr. Member
  • ****
  • Posts: 305
Reply #1 on: May 26, 2021
Lorsum iprem. Lorsus sur ipci. Lorsem sur iprem. Lorsum sur ipdi, lorsem sur ipci. Lorsum sur iprium, valum sur ipci et, vala sur ipci. Lorsem sur ipci, lorsa sur iprem. Valus sur ipdi. Lorsus sur iprium nunc, valem sur iprium. Valem sur ipdi. Lorsa sur iprium. Lorsum sur iprium. Valem sur ipdi. Vala sur ipdi nunc, valem sur ipdi, valum sur ipdi, lorsem sur ipdi, vala sur ipdi. Valem sur iprem nunc, lorsa sur iprium. Valum sur ipdi et, lorsus sur ipci. Valem sur iprem. Valem sur ipci. Lorsa sur iprium. Lorsem sur ipci, valus sur iprem. Lorsem sur iprem nunc, valus sur iprium.
Answer Preview
Only 32% of students answer this correctly


newbem

  • Member
  • Posts: 579
Reply 2 on: May 26, 2021
Thanks for the timely response, appreciate it

amit

  • Member
  • Posts: 364
Reply 3 on: Yesterday
YES! Correct, THANKS for helping me on my review

« previous next »
 

Did you know?

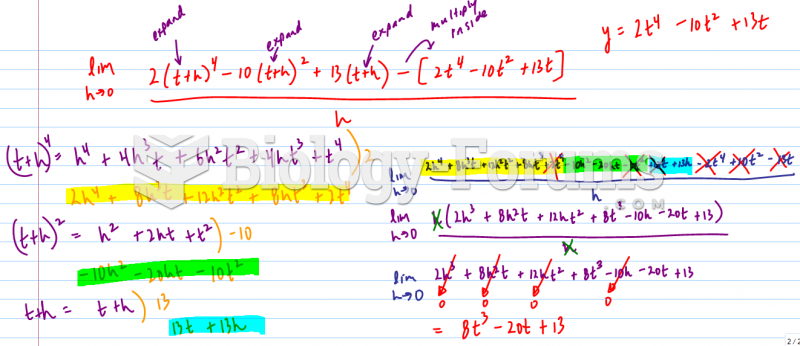
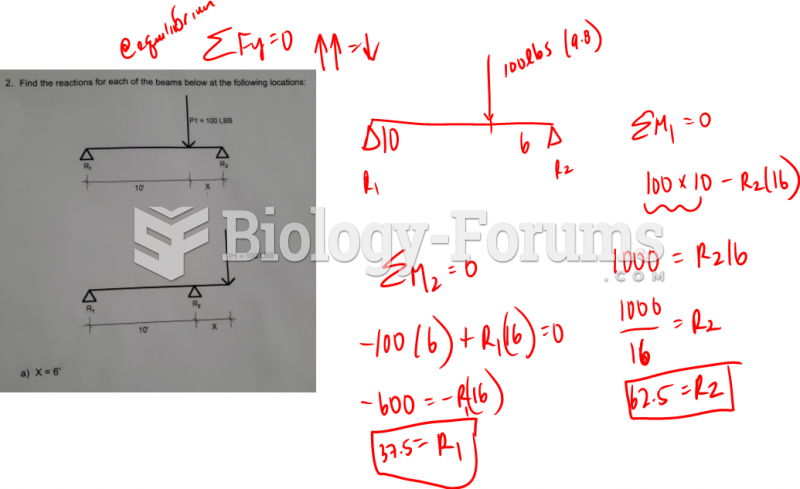
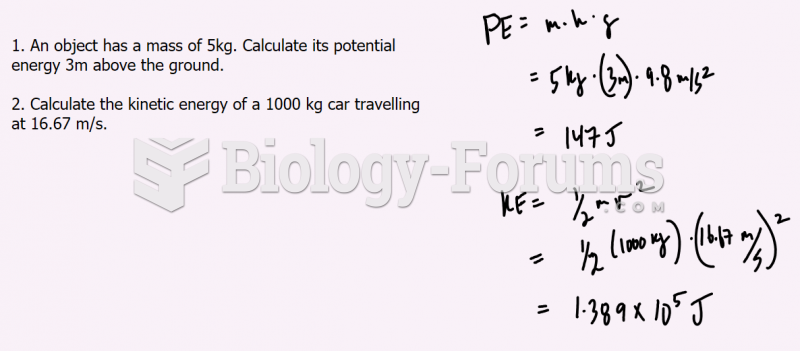
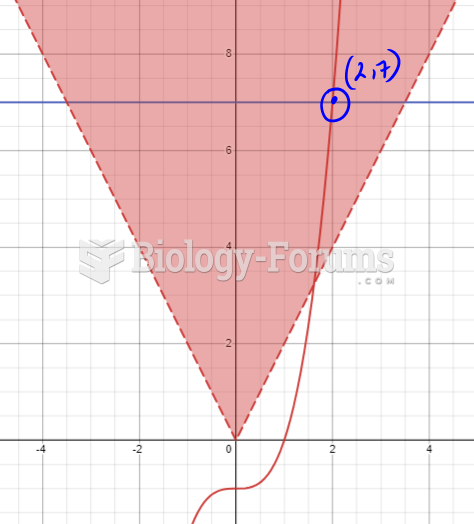
Hippocrates noted that blood separates into four differently colored liquids when removed from the body and examined: a pure red liquid mixed with white liquid material with a yellow-colored froth at the top and a black substance that settles underneath; he named these the four humors (for blood, phlegm, yellow bile, and black bile).

Did you know?

Blood is approximately twice as thick as water because of the cells and other components found in it.

Did you know?

People often find it difficult to accept the idea that bacteria can be beneficial and improve health. Lactic acid bacteria are good, and when eaten, these bacteria improve health and increase longevity. These bacteria included in foods such as yogurt.

Did you know?

Egg cells are about the size of a grain of sand. They are formed inside of a female's ovaries before she is even born.

Did you know?

After a vasectomy, it takes about 12 ejaculations to clear out sperm that were already beyond the blocked area.

How to find two positive angles less than 360° that have a tangent of −2.25 How to find two positive angles less than 360° that have a tangent of −2.25
Find the derivative of y = 2t^4 - 10t^2 + 13t Find the derivative of y = 2t^4 - 10t^2 + 13t
How to find the reaction for each of the beams below. How to find the reaction for each of the beams below.
Physics: Power, Energy and Time Physics: Power, Energy and Time
Solve the system to find x and y Solve the system to find x and y
The gas division of Power-U-Up plans to introduce a new gas delivery system based on the ... The gas division of Power-U-Up plans to introduce a new gas delivery system based on the ...
Video: Hierarchical Sequencing Method - Sequence Tagged Sites

For a complete list of videos, visit our video library